How to Show a Function Is Non Decreasing
In simpler words take two x-values on an interval. If the function value at the first x-value is less than or equal to the function value at the second then the function is said to be non-decreasing.
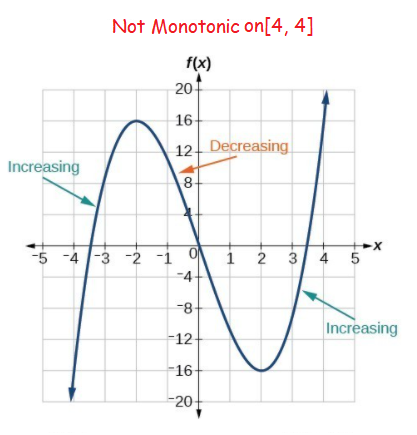
How To Determine If A Function Is Monotonic
F a1 f a1 f a1 Behaviour of f at a1.

. Thus overall we can say that so it is obvious for increasing or non-decreasing functions fx 0 with equality holding in interval like BC. Given an array A of size N 2 the task is to construct the array B of size N such that. F x P X x F x is bounded below by 0 and bounded above by 1 because it doesnt make sense to have a probability outside 0 1 and that it has to be non-decreasing in x.
The bonds sell at a price of 135354 and the yield curve is flat. Ii The function f is decreasing if and only if f x 0 for all x in I. Conversely a function fx is said to be nonincreasing on an interval I if fba with ab in I.
The dual terms are strictly decreasing and non-increasing reverse the direction of the inequalities respectively. The important parts are the and signs. A non-decreasing function is sometimes defined as one where x1 x2 f x1 f x2.
B f is of bounded variation on D. L f x 0 x a b For function to be non-increasing in. For a given function y F x if the value of y is increasing on increasing the value of x then the function is known as an increasing function and if the value of y is decreasing on increasing.
The usual way of proving that a function is non-decreasing is to analyze the sign of its first derivative. Increasing and Decreasing Functions in Calculus. A non-decreasing function f is one where x 1 x 2 f x 1 f x 2.
In calculus derivative of a function used to check whether the function is decreasing or increasing on any intervals in given domain. A function fx is said to be nondecreasing on an interval I if fbfa for all ba where ab in I. Remember where they go.
We summarize the results in the table below. To show that the function is non-decreasing I prefer the term weakly increasing this follows from the fact that the sum of weakly increasing functions is weakly increasing and that chi_ainfty is weakly increasing since if x. Let us see examples of each case.
Let fx dfraca frac1kbxsc lambda dx where acdklambda. I ℝ be a function. About Press Copyright Contact us Creators Advertise Developers Terms Privacy Policy Safety How YouTube works Test new features Press Copyright Contact us Creators.
If the function value at the first x-value is less than or equal to the function value at the second then the. Yield to Call Yield to Maturity and Market Rates Absolom Motors 14. Below is the graph of a quadratic function showing where the function is increasing and decreasing.
If fa fb for all a b. Refer to the graph in below-given figure b Only Increasing or non-decreasing functions- A function is said to be non-decreasing if for as shown in the graph for AB and CD. A strictly increasing function f is one where x 1 x 2 f x 1 f x 2.
B1 are some positive constants compare these letters with terms depending on m in the original function. A function f is said to be monotonic in an interval if it is either increasing or decreasing in that interval. Yield to Call Yield to Maturity and Market Rates Absolom Motors 14 coupon rate semiannual payment 1000 par value bonds that mature in 30 years are callable 5 years from now at a price of 1050.
ℝ ℝ such that f f1 f2 on D Dom f andVar Df Varf 1 Varf 2. When x1 x2 then f x1 f x2 Strictly Increasing. That has to be true for any x 1 x 2 not just some nice ones we might choose.
If a function changes its signs at different points of a region interval then the function is not monotonic in that region. There are two bounded non-decreasing functions f1 f2. F x is known as non-decreasing if f x 0 and non-increasing if f x 0.
A function is said to be increasing in the region where the value of the function y increases as we increase the value of x. Since my answer cant be deleted I update it to clarify the argument. My point is that this function cant be decreasing.
For a function yf x. If we draw in. A non-decreasing function is also defined as the one in which x1 x2 fx1 fx2.
L f x 0 x a b For function to be decreasingstrictly decreasing in. C There ane bounded non-decreasing functions f1 f2. Since your function is continuous and has no singularity you just need to compute F and observe that it can never be negative.
If their corresponding outputs decrease then the function is decreasing. L f x 0 x a b For function to be increasingstrictly increasing in. Find the non decreasing order array from given array.
Monotonically Increasing Increasing Non-Decreasing. When x1 x2 then f x1 f x2 Increasing. And for the portion BC.
Roughly given a function f it will be non-decreasing if fxge 0. If you have a quantity X that takes some value at random the cumulative distribution function F x gives the probability that X is less than or equal to x that is. So is decreasing while is increasing.
To be consistent lets assign as the smaller value the one furthest left on the graph. I The function f is increasing if and only if f x 0 for all x in I. ℝ ℝ such that f f1 f2 on D Dom f.
We can clearly see the blue graph it is that of the locus of the function f xy ax3bx2cxd. Lets show that the function fx 4x 3 6x 2 3 is decreasing between 0 and 1. Therefore the function is decreasing over.
Array A is given in such a way that the answer is always possible. Increasing and decreasing functions. A function is said to be decreasing in the region where the value of the function y decreases as we increase the value of x.
A i B i B n i 1. B is sorted in non-decreasing order. L f x 0 x a b.
In other words take two x-values on an interval. So So we can see that the x values are increasing. If fa fb for all a b.

Increasing And Decreasing Functions Geeksforgeeks

Increasing And Decreasing Functions

Math Teacher Mambo Math Teacher Calculus Positivity

Increasing And Decreasing Functions Geeksforgeeks
Ex Restrict The Domain To Make A Function 1 To 1 Then Find The Inverse Youtube

Pin By Frank Ligtenberg On D D D D Dungeons And Dragons Dungeons And Dragons Game Dnd Funny

Increasing And Decreasing Functions

Inverse Relations Lesson Algebra Algebra Lessons Algebra Worksheets

Monotonic Function Definition Examples Video Lesson Transcript Study Com
Monotonically Increasing And Decreasing Functions An Algebraic Approach Opencurriculum
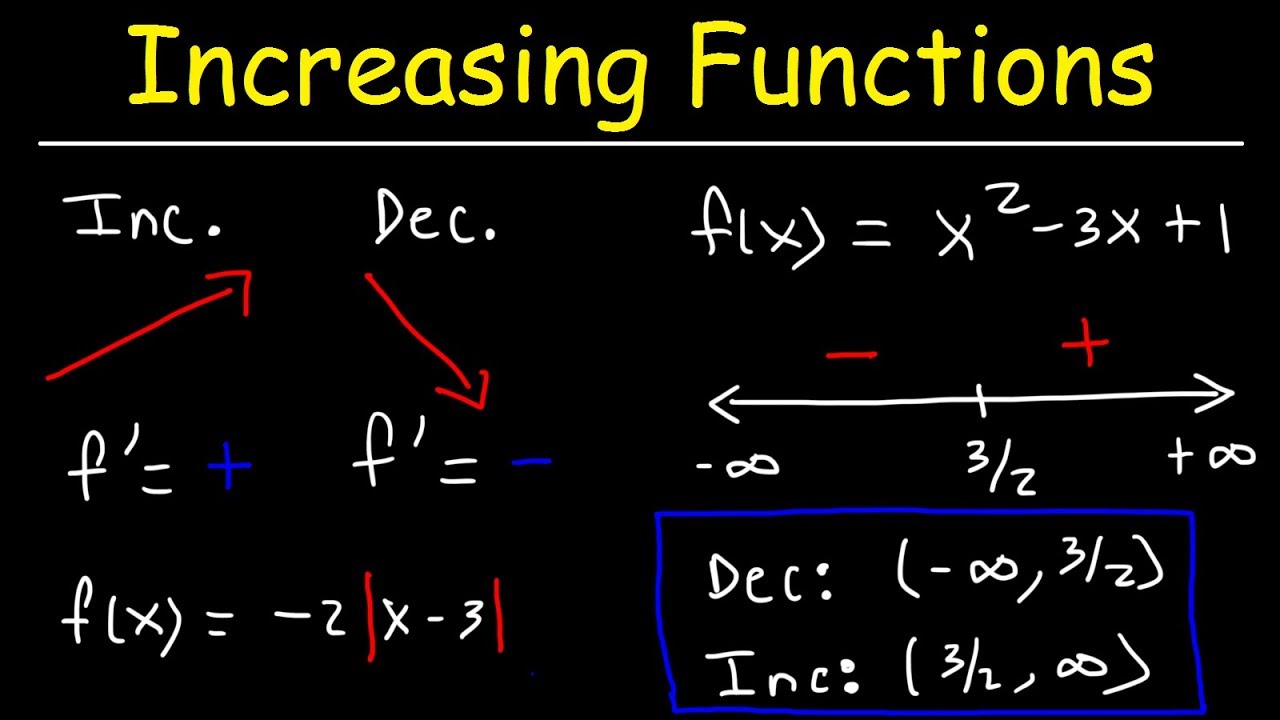
Increasing And Decreasing Functions Calculus Youtube

Monotonic Function From Wolfram Mathworld

Linear Proportional And Non Proportional Relationships Foldable Assessment Proportional Relationships Linear Relationships Math Interactive Notebook

Increasing And Decreasing Functions

Increasing And Decreasing Functions

Trigonometry Mazes 14 Mazes Law Of Cosines Trigonometric Functions Inverse Functions


Comments
Post a Comment